
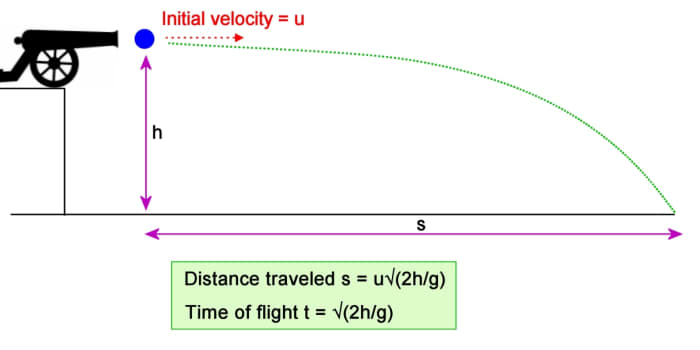
To find the formula for the range of the projectile, let's start from the equation of motion. There we have it: we figured out time, we figured out the average velocity, and so now we can figure out the height of the cliff. Launch from the ground (initial height = 0) The object’s maximum height is the highest vertical position along its trajectory.

Y h + V y x t g x t 2 / 2 In the above equation, h is the height of the object from where the object is thrown, t is the time, and g is the gravitational acceleration. Therefore, in a projectile motion, the Horizontal Range is given by (R): H o r i z o n t a l R a n g e ( R) u 2 sin 2 g Maximum Height of Projectile After understanding what a projectile is, let us know the maximum height of the projectile. Air resistance is neglected in all calculations.ġ. To determine the distance of the vertical components of the velocity for projectile motion can be written as. The launch velocity of a projectile can be calculated from the range if the. The equation for the height of a projectile, y (m). Include: horizontal and vertical components of motion of the. The basic motion equations can be solved simultaneously to express y in. Question: A common situation dealt with in engineering and physics is that of projectile motion. If you want to find the range for horizontal speed only (so angle = 0), you can use this projectile range calculator or go directly to horizontal projectile motion calculator page. Solve simple free-fall problems using the special equations for constant acceleration.
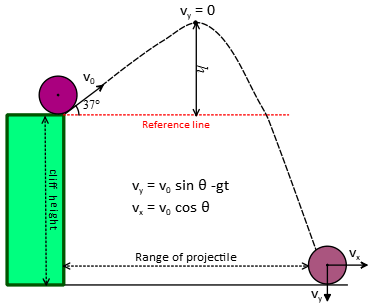
Immediately we spot that the final velocity, $v$ and the time, $t$ are not in SI units.Let's split the equations into two cases: when we launch the projectile from the ground and when the object is thrown from some initial height (e.g. It is the total time for which the projectile remains in flight (from O O to A A ).


 0 kommentar(er)
0 kommentar(er)
